Understanding Lumped Element Filters
Urvashi Sengal, Applications Engineer
Giri Krishnamurthy, Principal Design Engineer
The Basics: What is a Filter?
Before discussing filter designs and differentiating any given filter topology from another, it’s important to review the fundamentals of filter structures and their function. A filter is a two-port, passive, reciprocal device that allows frequencies within a given band to pass through while blocking signals outside the desired band.
There are many filter types available to the system design engineer including RLC filters, active RC filters, crystal filters, cavity filters, ceramic resonator filters and SIW, SAW and BAW filters. Filters may be fabricated using lumped elements, thin and thick film microstrip and stripline, LTCC and other manufacturing technologies. This article will focus on lumped element filters.
A Brief Review of Filter Theory
Filters are linear circuits that can be represented as a transfer function of the form shown in Equation 1, which corresponds to the simple block diagram in Figure 1.
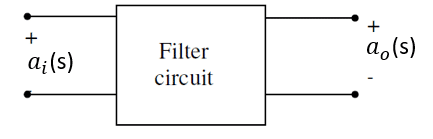
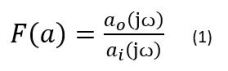
The transfer function F(α) describes the amount of energy lost through an internal filter circuit. F(α) is a complex number with both a magnitude and phase, and thus provides a mathematical representation of the network’s frequency response characteristics. The full gamut of filter transfer functions represents decades of research by industry and academia, but fortunately, filter designers today have the advantage of obtaining and modelling transfer functions with commercially available filter synthesis software.
The transmission of any filter can be characterized with the following parameters:
Passband Frequency (ωp) – The range of frequencies that can pass through a filter
Stopband Frequency (ωs) – The range of frequencies that the filter rejects or attenuates
Insertion Loss – The maximum allowed attenuation in the passband
Stopband Rejection – The minimum allowed attenuation in the stopband
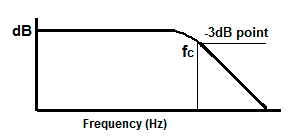
Cut-Off Frequency (fo) – The frequency at which filter insertion loss is equal to 3 dB
Center Frequency (fc) – The frequency at which band-pass filters are geometrically centered. For example, if f1 and f2 represent the 3 dB frequency points of a band pass filter, then the center frequency fc is calculated as follows:
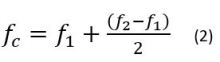
Sharpness (or Selectivity) – The steepness of the transition between passband and stopband.
Group Delay – A measure of a device’s phase distortion within the passband. Ideally, all frequency components of the input signal are shifted (usually delayed) in time by the same constant amount.
Lumped Element Filter Characteristics
Circuit analysis typically assumes that the physical dimension of a network is much smaller than the electrical wavelength of the incident signal. Voltages and currents do not vary with the physical dimensions of lumped elements in the filter. Lumped element filters are passive, lossless and reciprocal, and consist of inductors (L) and capacitors (C). The generic structure of a lumped element L-C filter is shown in Figure 2 below.
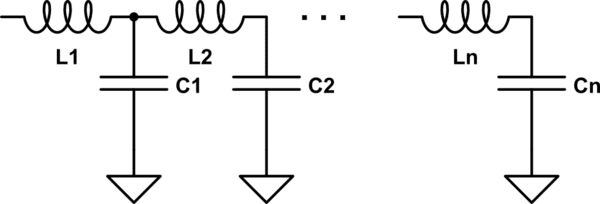
Lumped element filters are basic building blocks for other, more complex filter designs. Lumped elements can be transformed for compatibility with multiple modern filter technologies such as microstrip, stripline, LTCC, MMIC and others.
A filter shapes the frequency spectrum of the input signal according to the magnitude of its transfer function. The phase characteristics of the signal are also modified from the filter’s input to output. Filters can be classified into several categories based on the frequency bands they pass and those that they block. The four primary filter response types are shown each in turn below.
Low Pass Filter
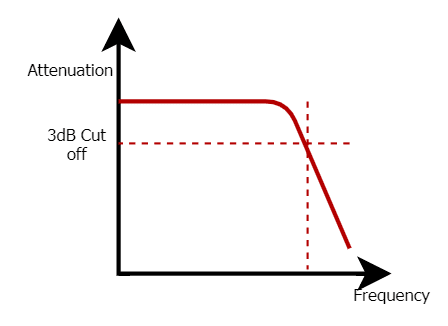
As the name suggests, a low pass filter allows only low frequencies to pass through while rejecting high frequencies. High frequencies are attenuated above the 3 dB cut-off as shown in Figure 3.
High Pass Filter
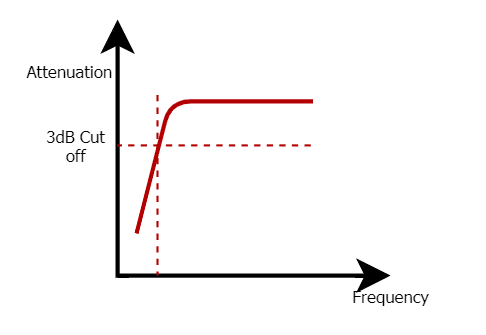
A high pass filter has the opposite response to that of a low pass filter. This filter type allows high frequency components above its 3 dB cut-off point to pass through while blocking low frequency components.
Band Pass Filter
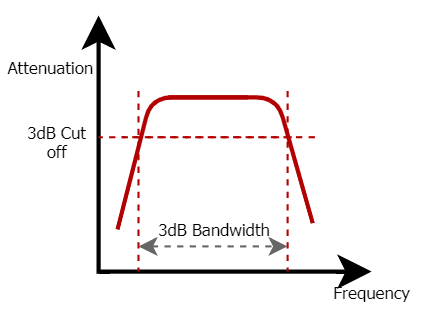
A band pass filter allows signals within a designated frequency band to pass through, while rejecting signals above and below 3 dB cut-off points at the upper and lower passband edges.
Band Stop Filter
Band stop filters have the opposite response to that of a band pass filter, rejecting signals within a designated frequency band while passing signals above and below 3 dB cut-off points at the upper and lower edges of the stopband.
Basic Properties of Lumped-Element Low Pass Filters
An ideal low pass filter should pass all signals with frequencies lower than the cut-off frequency and attenuate all signals with frequencies higher than the cutoff frequency. The level of attenuation for each frequency depends on the filter design and order. We don’t live in an ideal world, however, and an ideal filter does not exist in practice. The performance of a filter will always be affected by factors such as dielectric loss and the conductivity of metallic materials used in the circuit.
For example, if the most valuable performance attribute to a designer is maintaining minimum insertion loss across the desired band, then a maximally flat filter response should be implemented. If a sharper cut-off in the transition is needed, on the other hand, then a Chebyshev response would be preferable.
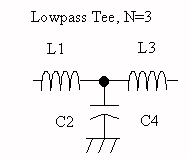
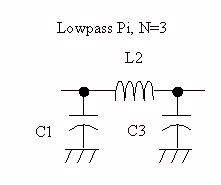
In a lumped element filter, each capacitor and inductor pair represent a pole or “zero” in the frequency response. When an input signal is applied to a filter, the output is a composite of present inputs with past inputs and outputs. These past signal components represent nothing but the delay. Maximum delay is the calculation of all outputs and is a function of the order of the filter circuit.
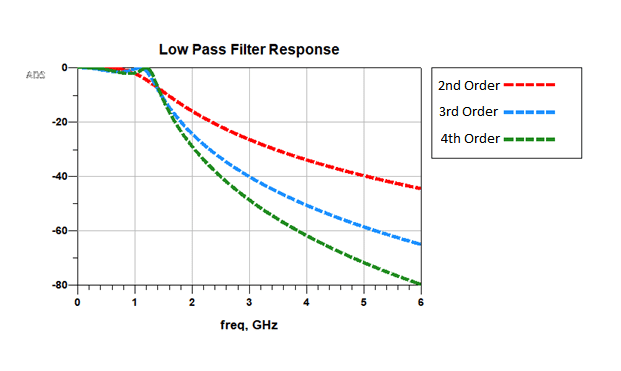
In the T and Pi filter networks shown in Figure 6, three delay elements are present, so the order of the filter N = 3. Adding more elements to a filter can increase its roll-off (or sharpness), as shown in Figure 7.
Any type of filter response can be derived from a low pass structure using frequency and impedance scaling as shown in Figure 8 below, where L and C represent the impedance-scaled element values of the normalized low-pass prototype of order n.
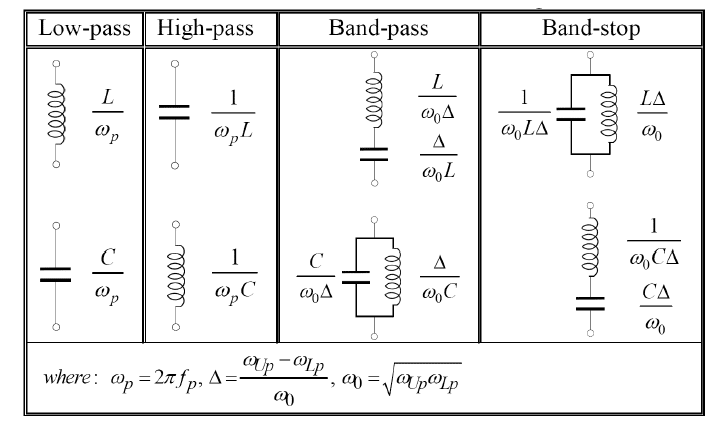
Lumped Element Filter Topologies
There are four major types of responses to consider when designing L-C filters:
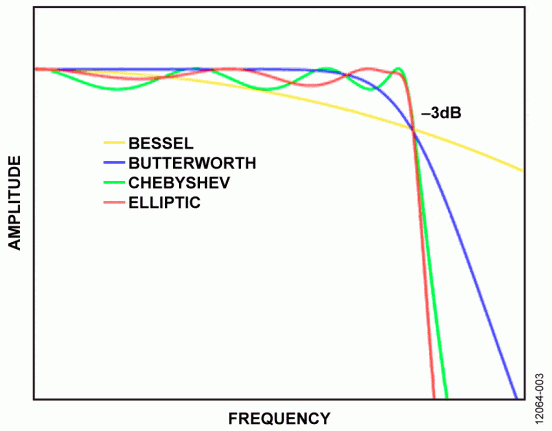
Maximally Flat Response / Butterworth
This is also called a monotonic response, based on the Butterworth polynomial. As the name suggests, it provides a flat passband response for a given filter complexity. For , attenuation increases monotonically with frequency.
Chebyshev Response
This characteristic is also referred to as the Equal Ripple Response, based on the Chebyshev polynomial. It provides some ripple in the passband but higher attenuation in the stopband. Insertion loss for Chebyshev is greater than that for a maximally flat response for a given frequency where ω >> ωc.
Elliptic Response
This function exhibits ripple in both the passband and the stopband. Many applications require a certain level of attenuation in the passband and stopband. In this case, a better cut-off can be achieved with the elliptic function.
Bessel (Linear Phase)
This function provides maximum flatness in group delay and preserves the shape of signals within the passband.
It should be noted that, in addition to these four response types, practical filter design employs several variants of these topologies to arrive at a desired response.
Advantages and Disadvantages of Lumped Element Filters
Lumped element filters offer many advantages. They work well with lower frequencies, and are capable of achieving bandwidths from 10 percent to 90 percent. These filters provide sharper responses with the use of air coils, which offer a higher-Q. Lumped element filters are relatively easy to design and tune if they are physically smaller than the operating wavelength. In addition to easy design and tuning, L-C filters are easy to build and require minimum tooling cost, which gives a high degree of freedom for customization.
However, as with most things, lumped element filters also come with some disadvantages worth noting. It is difficult to achieve very narrow bandwidths with these filters, and the parasitics are often unpredictable at high frequencies. This makes them impractical to fabricate at high frequencies. In addition, lumped inductors and capacitors are generally only available for a limited range of values and cannot handle very high power. At lower frequencies (kHz), inductor size is generally too bulky and hard to design.
Advantages of L-C Filters
Disadvantages of L-C Filters
- Works well with lower frequencies
- Smaller footprint compared to other technologies like cavity filters
- Capable of 10% to 90% bandwidth
- Sharper response by integrating air coils
- Easy to design and tune
- Easy to build with minimum tooling cost
- High degree of freedom for customization
- Difficult to achieve narrow bandwidths
- Parasitics unpredictable at higher frequencies
- Impractical to fabricate at high frequencies
- Lumped L and C generally only available for limited range of values
- Cannot handle very high power
- Bulky and hard to design at very low frequencies (kHz)
Conclusion
Mini-Circuits is constantly pushing the envelope for lumped element filters in terms of power, frequency, miniaturization and RF performance, all with little to no impact on the cost to the customer. L-C filters represent a major group within the company’s vast filter portfolio, offering customers a wide range of standard and custom options for their application requirements.
Some of the latest designs over high power handling up to 20, 40 and even 100W. Other models now offer stopband rejection up to 60 dB or better. The ULP-series offers one of the smallest form factors in the industry (0.25 x 0.25 x 0.1”). Filters are available in connectorized and surface mount housings, as well as newly developed packages resistant to aqueous wash.
For additional support finding the ideal lumped element filter for your needs, reach out to one of our engineers.
View all RF Filters